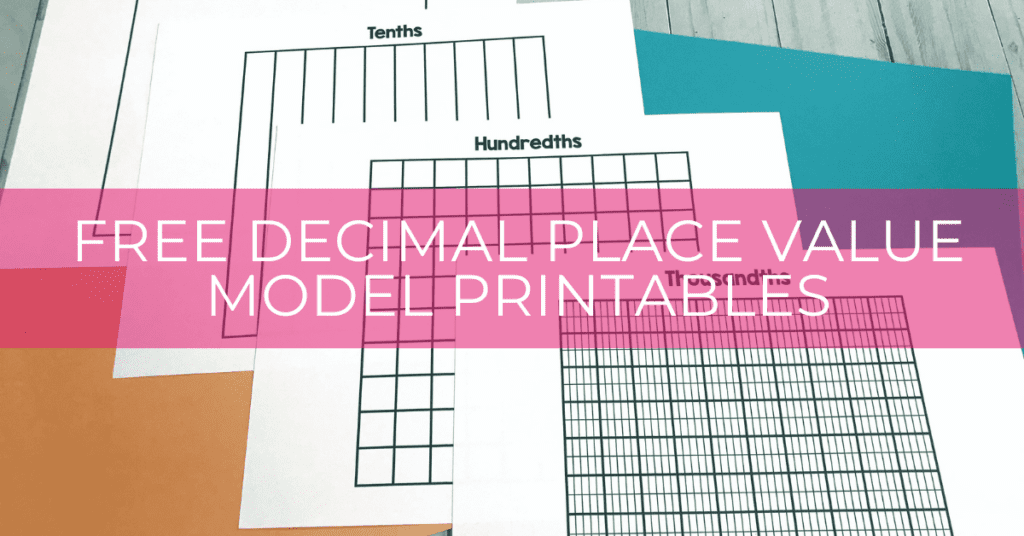
Completely understanding decimals can be incredibly tricky. We are asking kids to begin to understand quantities that are less than one. There are many mistakes I see students make when working with decimals. Teaching place value of decimals in a way students understand is essential.

Decimal Place Value Mistake #1: The ‘Oneths’ Place
Ok, WE know that the “oneths” place doesn’t exist. It’s not a thing. The problem is that students don’t know this. Once students notice that the decimal place value positions mimic the whole number positions, they may falsely believe there is a ‘oneths’ place. This misunderstanding comes from thinking that the positions reflect over the decimal point. In reality, they reflect over the ones place.
To help students see this, I show them a list of just the place value positions, but without any numbers or the decimal point. We talk about patterns they notice and help them draw conclusions about the organization of the place value positions.
Decimal Place Value Mistake #2: Reading the Decimal
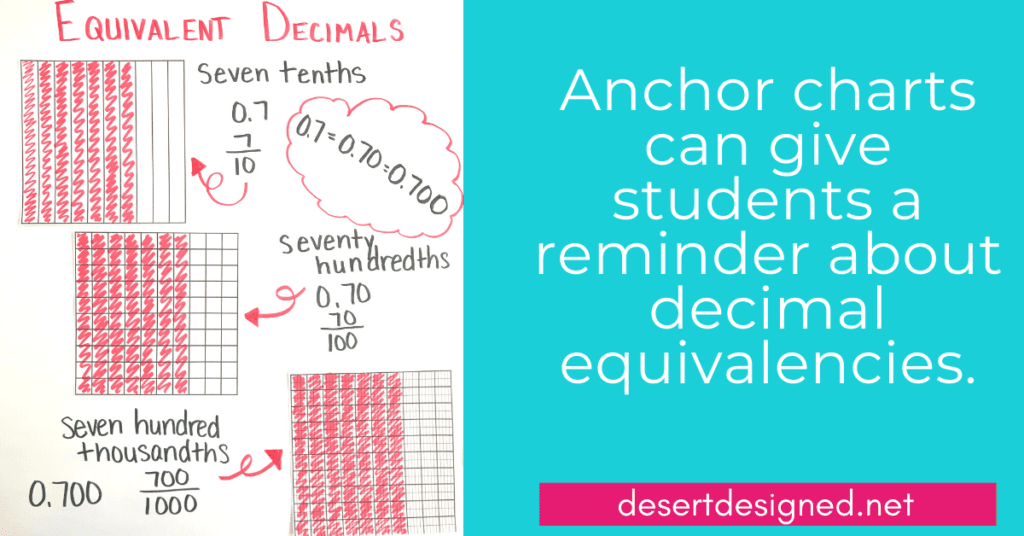
In my time as an upper elementary teacher, I have heard students try to name decimals in a large variety of ways. Let’s take this number as an example:
6.304
I have heard this read as:
- Six decimal three oh four
- Six thousand, three hundred and four
- Six-point three zero four
- Six-point three hundred four thousandths
As a teacher, this can feel frustrating. We need to realize that students are doing what they can to describe the number they see before them. They get several of these descriptions from us as teachers. When it is time to read numbers to kids, particularly decimal numbers, we often quickly say something like ‘six-point two’ instead of ‘six and two tenths.’
Our intention is genuine. We need students to write six point two quickly. It is efficient, appropriately descriptive, and there is nothing wrong with it. Except, if you want your students to understand decimal place value, it does not reinforce what we teach.
How do we fix this? Begin with teaching students to read the decimal numbers methodically.
Here is the method I use:
Step 1: Read the number before the decimal point as if the decimal numbers are not there. (For students who struggle, I use my hand or a post-it to cover up the decimal place value positions)
Step 2: For the decimal point, say “and”
Step 3: Read the number after the decimal point as if the decimal is not there. (Once again, if students struggle, I cover up the beginning of the number)
Step 4: Finally, say the place value position of the final digit in the number.
By using this method, our sample number becomes:
Six and three hundred four thousandths.
Teaching place value of decimals through correct word form will help students greatly in the long run. I love these Decimal Word Form Boom Cards to help students practice.

Decimal Place Value Mistake #3: Reversing the Place Value Positions
Students struggling to grasp the place value of the digits will sometimes reverse the place value positions within the decimal places. For example, since hundreds are greater than tens, students may assume that hundredths are greater than tenths.
This mistake comes from a lack of understanding of the quantity in those place value positions.
To tackle this misunderstanding, we can use models to help students see the connection between the place value words and the value of the digits. There are several types of models you can use to represent place value. My two favorite types of models for this are place value blocks and paper “hundredths squares.”
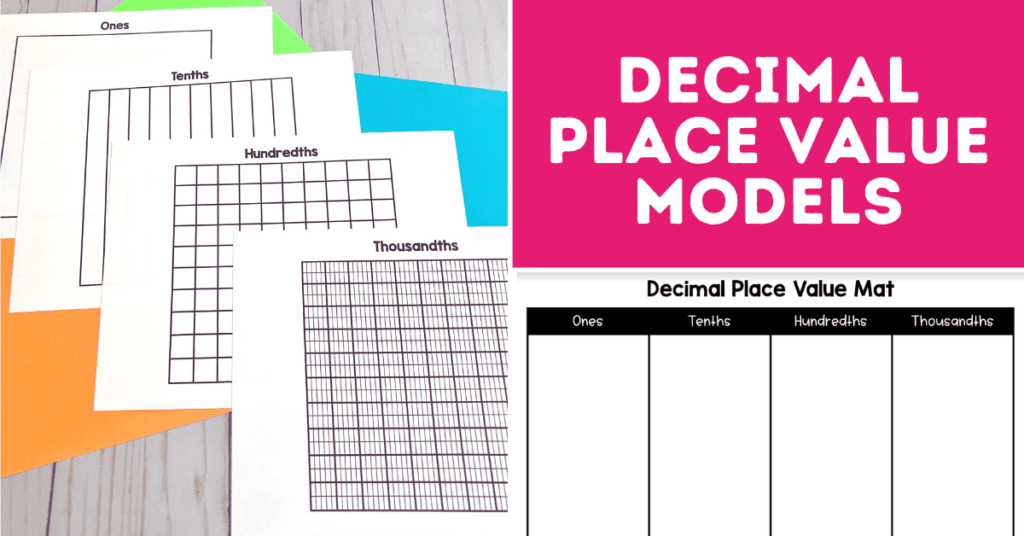
To learn more about using place value blocks and hundredths squares as models for decimal place value, make sure to check out my blog post about teaching models for place value of decimals for more explanation and ideas!
Decimal Place Value Mistake #4: Seeing the Decimal as a Comma
The mistake of seeing the decimal point as a comma is less common but still occurs. Students who lack foundational place value skills will sometimes confuse the symbols or view the decimal as another comma within the number.
This misconception is most common in students who struggle with the correct placement of commas in whole numbers. These students need continued practice with place value skills both in the whole numbers and in decimals.

You can provide this practice of correctly writing the numbers by utilizing place value charts, models, and repeated practice. It would be best if you didn’t allow students who are making this mistake to practice the mistake repeatedly. These students should attend small groups, work with a partner to repeatedly check the accuracy of their comma and decimal placement, or practice writing and reading numbers on digital platforms such as with these cards on Boom Learning.
Decimal Place Value Mistake #5: Inflexibility when Using Models
Many students become confused when we transition place value blocks from representing whole numbers to representing decimals.
When using place value blocks with students to represent decimals, it is crucial to explain explicitly to the class that they will be using the models to represent different quantities than they have previously experienced. They need to be flexible in thinking about different pieces representing the one from this point on.
You can help them with this by doing the following activity:
The first student decides which place value piece will represent the “one.”
Then, the other students create a number on a place value mat using place value blocks and write the number correctly.
(photos of this in action)
Click here to join my email list and get a free copy of this place value mat for easy download.
When students realize that the quantities assigned to each representation can be different, it will strengthen their understanding of place value and how different place value positions relate to one another.
Decimal Place Value Mistake #6: Comparing Decimals
Students’ misconceptions about decimal place values become most apparent when they start comparing decimals. A student with a firm grasp of place value will compare decimals accurately and with ease. However, students who still struggle with the foundations of decimal place value will have a tough time with this task.
Having a student compare two decimals and explain their comparison to you is a great way to see if they genuinely understand decimal place value. You can learn a lot about how they think about decimals and the value of the decimal positions by their explanation.
To test their understanding, you want to give them a problem similar to this:
Which is greater: 0.39 or 0.9
A student who understands place value will be able to articulate that 0.9 is greater. There are many ways they can explain this. For example:
- Nine tenths are greater than three tenths, so 0.9 is greater.
- Ninety hundredths are greater than thirty-nine hundredths.
- Having nine dimes is better than having three dimes.
- If students understand the relationship of each number to one whole, the student may tell you that 0.9 is closer to one than 0.39.
A typical explanation from a struggling student or a student who does not yet have a full grasp of decimal place value would be that thirty-nine is greater than 9, so 0.39 is greater.
To help students overcome this, we should help students represent the quantity using paper models and place value blocks. Next, write the numbers into a place value chart and ask them what the different place values mean.
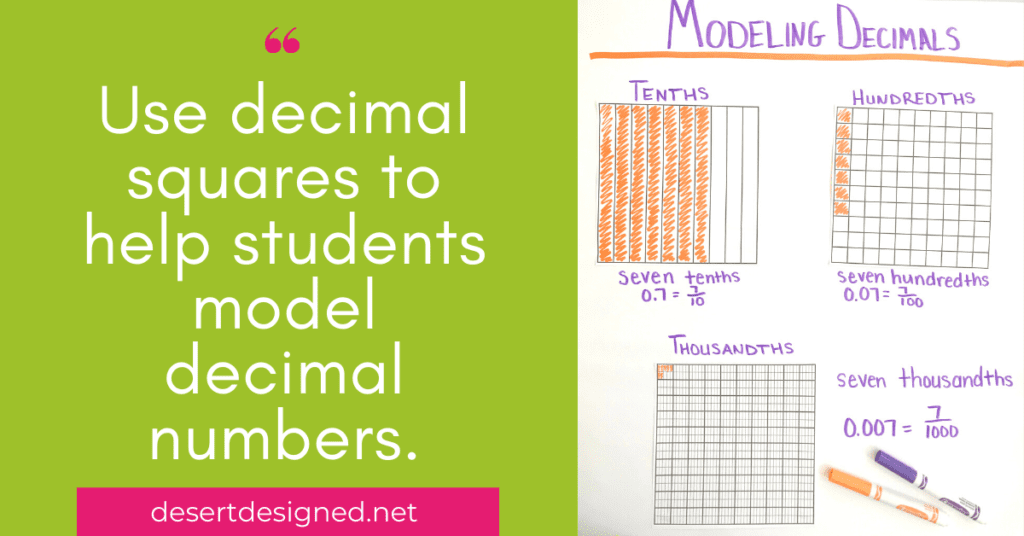
If this is a struggle with your class as a whole, before having students compare, spend time doing lessons that reinforce this concept. I love using this anchor chart in my classroom to help them compare the decimals in a way that reinforces the concept.
Practice, Spiral, and Reinforce
As teachers, teaching place value of decimals concepts happens early in the year and often fail to return to them later. Not returning to place value is one of the many problems contributing to difficulty retaining decimal place value concepts.
We must return to place value throughout the year and talk about the value of numbers in computation problems. Additionally, we need to reinforce using the correct place value vocabulary throughout the school year.
Using anchor charts in my classroom has helped me do this by giving me something to refer to throughout the year. I love using these hundredths squares to help me make models for my anchor charts that are easy for students to see from anywhere around the room. Don’t forget to sign up for my email list to get your set today!