Understanding standards as a teacher is half the battle. Multiplication as scaling had me baffled when I first saw this. This post will explore what it means and how to teach it to your students.
Kids and Teachers Can be Intimidated when they Hear Multiplication as Scaling.
According to Common Core, here is the text of the standard for multiplication as scaling that students must understand:
Interpret multiplication as scaling (resizing) by:
Comparing the size of a product to the size of one factor on the basis of the size of the other factor, without performing the indicated multiplication.
Explaining why multiplying a given number by a fraction greater than 1 results in a product greater than the given number (recognizing multiplication by whole numbers greater than 1 as a familiar case); explaining why multiplying a given number by a fraction less than 1 results in a product smaller than the given number; and relating the principle of fraction equivalence a/b = (n × a)/(n × b) to the effect of multiplying a/b by 1.
It sounds More Difficult than it is
When I first started teaching Common Core State Standards, I came across this doozy, and I was like….what?!?! It just didn’t make sense to me as I read it. I think the problem was that the standard is so wordy. I hadn’t previously heard anything like it despite teaching 5th-grade math for several years.

In reality, helping students understand multiplication as scaling isn’t all that difficult.
Multiplication “Always” Makes Numbers Larger
One of the first barriers to helping students interpret multiplication as scaling is that most students have learned multiplication always makes numbers larger. I can understand fully why teachers of lower grades teach this rule. As students are creating arrays, memorizing their division facts, and learning how to do more complex whole-number multiplication problems, we want them to assess the reasonableness of their answers. If a student multiplies 362 x 4 and gets 128, they have made a mistake.
However, this commonly repeated “rule” expires when students begin to multiply by fractions and decimals that are less than one.
Foundational Understanding of Decimal and Fraction Numbers
Students lacking a basic understanding of the quantity represented by a fraction and decimal numbers tend to struggle the most with this skill. Before approaching this standard, I highly recommend reviewing basic fraction and decimal concepts to ensure that students understand their quantities as less than one. If you need some tips for teaching decimals, check out our post for that here.
Multiplication as Scaling Means Students Understand Reasonableness of their Answers
Once students have mastered the understanding of interpreting multiplication as scaling, they are much more easily able to self-assess the reasonableness of their answers. Reasoning about fraction multiplication is crucial for students as the next step into more complex mathematical reasoning is necessary for future years.
Helping Students Understand Multiplication as Scaling
Isolate the Multiplicand
To help students begin to understand multiplication as scaling, I highly recommend that you repeatedly use the same multiplicand. By repeating one factor in a variety of problems, students can see the changes to the number as it is multiplied by a variety of different numbers to scale.
Use Kid-Friendly Terminology
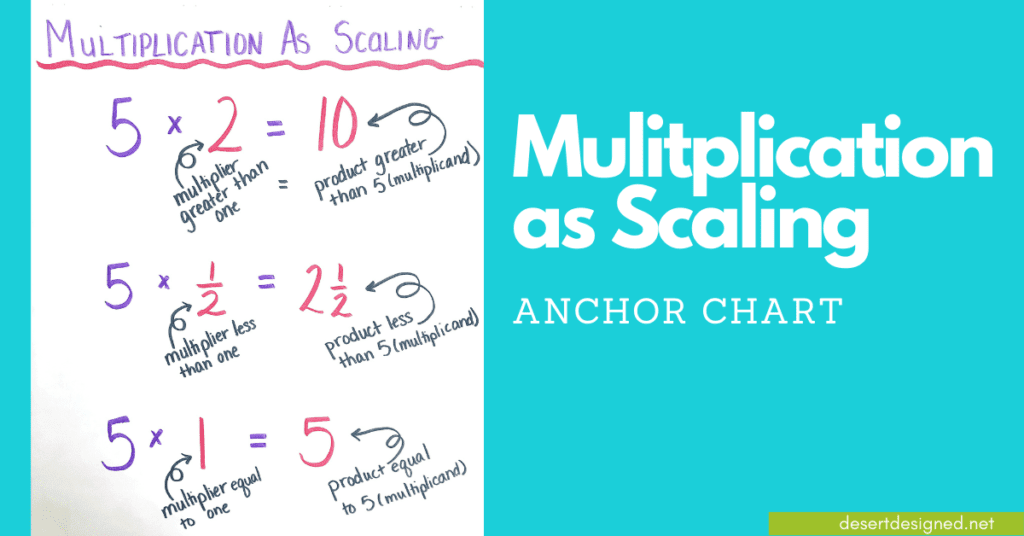
In addition to repeating the same factor multiple times, use kid-friendly terms to help students think about what is happening in the multiplication problem. Focus on one factor. For example, in 5 x ½, assess the problem by comparing the answer to the five. The product will be smaller than five since it was multiplied by one-half. I teach my students that the ½ causes what it is being multiplied by to “shrink.” Some other ways you could present it is that the amount of the other number will decrease, get smaller, lessen, or reduce.
For the next problem, we will multiply 5 x 2. Kids already know this math fact, but we focus on what happens to the product compared to the 5. In this case, we talk about the product as “growing.” Some other terminology you could use is to increase, enlarge, or expand.
Using a simple fraction and a whole number is pretty straightforward. After addressing those types of numbers, we also look at what happens when we multiply a number by a mixed number or improper fraction. The key here is to focus on the value of the multiplier and how that multiplier affects the other factor. For example, multiplying 5 x 7/2 will cause the 5 to grow because 7/2 is greater than one.
Finally, students need to recognize what happens when multiplying any number by one or a fraction equivalent to one. By fifth grade, most students are competent in knowing that numbers stay the same when multiplied by one. However, we need to be sure to address fractions that are equal to one as well. Some examples are 2/2, 3/3, 4/4, and so on.
Use Kinesthetics to Demonstrate
After examining what happens to numbers multiplied by multipliers of various sizes, I love doing this activity. It helps to build the understanding for students that they can interpret the multiplication as scaling without having to find the actual products.
In this activity, I pass out a piece of paper with a large number written on it to all students. I tell my students that they have assumed the identity of the number I have given them. Next, I tell them that they are being multiplied by a given multiplier.
If they have grown, they stand up out of their chairs. Students who shrink squat or sit down. If their value has stayed the same, they are to remain seated.
To share the numbers with them, I write them on large paper or share them in a PowerPoint, one multiplier at a time.
The speed associated with this activity helps students calculate the problem on a paper to decide if the number increases, decreases, or stays the same.
Take Some Notes
After lots of practice, have students record notes related to the effects of multiplying a number by fractions or decimals. Often, I have students take notes at the beginning of a math topic. However, in this particular standard, I wait until we have worked through various problems and practiced for some time before taking notes.
The wording to explain this concept in writing can be complicated (as indicated by the crazy-long text of the standard). That longer wording can feel overwhelming for students as they are introduced to this standard and cause many students to shut down and pre-determine that they won’t understand. As a result, I now have students take these notes AFTER they understand the concepts.
One way to truly help them lock in this understanding is to solicit students to help you craft the wording of these notes rather than just giving it to them!
Practice Multiplication as Scaling
Usually, once kids get the hang of this skill, it will come very quickly to them. However, coming to that understanding can take time and practice. I love using this Multiplication as Scaling Boom Cards Deck to help my students continue to practice this skill.



